Compound annual growth rate or CAGR – is the annually compounded rate of return. The rate at which investment should grow to reach an ending balance.
What is Compound Annual Growth Rate or Commonly Known as CAGR?
Compound annual growth rate or CAGR, as we commonly know the term – is the annually compounded rate of return. It is the rate at which investment should grow to reach an ending balance.
As an example, if you wish to double your money in 3 years, the CAGR returns have to be at ~26%. Similarly, if you want to double the money in 4 years, the return should be around ~19%. Don’t worry. We will see how to arrive at those rates soon.
CAGR is mainly a function of starting balance and ending balance.
Formula and Calculation of CAGR
The mathematical formula for CAGR is as follows:
If you wish to represent the rate in percentage terms, multiply it by 100.
Now taking the example I mentioned, if you wish to double your money in 3 years, the CAGR returns have to be at ~26%. So let’s understand how we get the rate as 26% and what does it mean in reality.
Example of How to Calculate CAGR
The initial amount is ₹100, and the final amount is ₹200 that we want at the end of 3 years.
So putting it in the formula, we get the result as follows:
= ((100/200)^(1/3))*100 = 25.992 or close to 26% CAGR.
In other words, if we wish to double the money in 3 years, we need a CAGR return of ~26%.
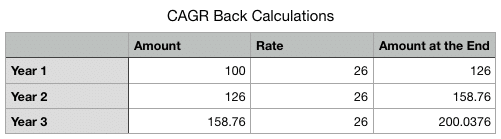
So what it means is our initial investment of ₹100 at the end of the first year should become ₹126. Now with ₹126 as the initial capital for the second year, and a return of 26% again. So 26% return on ₹126, and it becomes ~₹158. Applying the same on ₹158 at the end of the third year, we get our final double amount of ₹200.
We can apply the same for ~19% CAGR return and will see the money doubles in 4 years.
As a side note: If you wish to make 10x money in 10 years, your CAGR return should be very close to ~26%.
Real-Life Example of CAGR Calculations
In real life, you don’t always have the number of years as integers. So let me share a real-life calculation for my investment in Pidilite that I did on 08-Sep-2016. (Contract Notes attached in this article here).
For the number of years, we use the number of days and divide it by 365.
So between 8 September 2016 and 15 December 2019, there are 1,193 days.
So the number of years I am holding the investment becomes = (1193/365) = 3.27 Years.
The initial amount invested was for the thousand units was: ₹7,18,864
The final value of the investment as on 15th December 2019 is: ₹13,24,450
I was paid dividends three times in the interim, which is ₹4750, ₹6000, and ₹6500.
So the total final Amount is: ₹13,24,450 + ₹4750 + ₹6000 + ₹6500 = ₹13,41,700
= ((7,18,864/13,41,700)^(1/3.27))*100 = 21% CAGR.
The same CAGR is shown in the portfolio of ValueResearchOnline.
Why is CAGR important?
CAGR is one of the better ways to determine the average return rate for an investment. Moreover, the investor can compare the CAGR of two investment alternatives to evaluate one investment over others.
As an example, if you wish to 10x the money in 10 years, your return on investment should be ~26%.
Now compare that to Page Industries, which has given ~100x returns in the past ten years. The rate is over 58% CAGR.
Motherson Sumi has become 30x in 10 years at a CAGR return of 40%.
Comparing CAGR helps understand how an investment is performing as compared to others.
Limitations of CAGR
The most common limitation of CAGR is, it doesn’t take into account the amount we add in between or the amount we take out – only considers the initial investment and the final amount.
So in our example above, the dividend was paid out at the end of each year, but for the CAGR calculations, we only considered the final amount and not the days when it was taken out.
So, the above formula for CAGR return on SIP has to be modified where each investment has to have a different CAGR return based on the time the amount remained invested. It is one of the reasons why in ValueResearchOnline, we see the CAGR return as 21.20 as opposed to my calculation of 21%.
One more limitation of CAGR is, it can vary depending on when you start the calculation, and when you end the calculation.
Consider an investment of 1L in 2015, and in the next three years, the amount is as follows: 95k in 2016, 93k in 2017, and 92k in 2018. In 2019 if the investment shoots up to 125k.
If you consider the CAGR returns in 2019, your CAGR return is expected to be positive and close to ~6% with an assumption it was linear returns each year. However, it is not an accurate picture.
Still, the way to look at the number is, you made a CAGR return of 6% return on investment in the four years.
Final Thoughts
Every mathematical formula will have some limitations. Still, CAGR is very widely used, and one should rely on it to find the kind of growth companies can deliver over a longer time frame.





Leave a Reply